Geometry Sum | ABCD is a square. Prove in each case ∆APD ≅ ∆BPC | Find Angle DPC Angle PCD and Angle PCD |

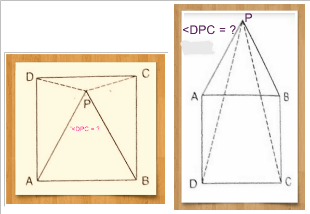
   ABCD is a square and APB is an equilateral triangle.
Prove in each case:
  1) &#x2206APD ≅ &#x2206BPC
   2) Find the angles of &#x2206DPC

Solution :
First Part
Given &#x2206ABP = Equilateral triangle
R.T.P.
1) &#x2206APD ≅ &#x2206BPC
   2) Find &#x2220DPC, &#x2220PDC and &#x2220PCD,
Proof: In &#x2206APD and &#x2206BPC

AP = BP | Same side of the equilateral triangle.
AD = BC | Same side of the Square.
and
&#x2220DAP = &#x2220 DAB – &#x2220PAB = 90^°-60^°=30⁰
Similarly
&#x2220BPC = &#x2220 ABC – &#x2220ABP = 90^°-60^°=30^°
∴ &#x2220DAP = &#x2220BPC
∴ &#x2206APD ≅ &#x2206BPC SAS_Proved

2nd Part

In &#x2206APD
AP=AD || As AP = AB (Equilateral Triangle).
We know that &#x2220DAP = 30 ^°
∴ &#x2220APD = (180^°– 30^°)/2 || &#x2206APD is an isoscless &#x2206 and &#x2220APD is one of the base angle.
or &#x2220APD = 150^°/2
or &#x2220APD = 75^°
Similarly
&#x2220BPC = 75^°
Therefore &#x2220DPC = 360^°– (75^°+75^°+60^°)
or &#x2220DPC = 150^°
Now in &#x2206 PDC
PD=PC || As &#x2206APD ≅ &#x2206BPC
∴ &#x2206PDC is an isocles &#x2206
And &#x2220PDC = &#x2220PCD = (180^° -150^°) /2
Or &#x2220PDC = &#x2220PCD = 15^°
∴ &#x2220 DPC=150^°
&#x2220 PDC=15 ^°
and &#x2220 PCD=15 ^° Answer

2nd Case proof for 2nd image and angle calculation are coming soon